Mathematicians


Matthias Bernegger (latin: Bernegerus or Matthew) was an Austrian and French scientist, astronomer, mathematician, linguist and translator.
He was educated in Strasbourg, where he developed a special interest in astronomy and mathematics. Bernegger corresponded with the famous scientists Johannes Kepler and Wilhelm Schickard. From 1607, Bernegger taught at the Strasbourg Gymnasium, and in 1616 he was appointed professor at the Academy.
Bernegger is known for his translations of Justinian and Tacitus, and in 1612 translated into Latin Galileo's 1606 work on the proportional compass, adding considerably to it. These additional detailed annotations by Bernegger made Galileo's compass much easier to use, making it the first mechanical calculating device that could be applied to a wide variety of complex problems. In 1619 Bernegger prepared a three-volume manual of mathematics, and in 1635 he translated Galileo's Dialogue on the Two Mass Systems of the World.


Jakob Bernoulli was a Swiss mathematician. One of the founders of probability theory and mathematical analysis. Johann Bernoulli's older brother. Proved a special case of the law of large numbers - Bernoulli's theorem. Professor of Mathematics at the University of Basel (since 1687). Foreign member of the Paris Academy of Sciences (1699) and the Berlin Academy of Sciences (1702).
Jacob Bernoulli contributed greatly to the development of analytical geometry and the birth of the calculus of variations. Bernoulli's lemniscate is named after him. He also investigated the cycloid, the chain line, and especially the logarithmic spiral. The last of these curves Jacob willed to be drawn on his grave; through ignorance, Archimedes' spiral was depicted there.
Jacob Bernoulli is credited with significant achievements in series theory, differential calculus, probability theory, and number theory, where the "Bernoulli numbers" are named after him.


Johann Hartmann Beyer was a German physician, mathematician and statesman.
He earned a master's degree in liberal arts at the University of Strasbourg, and then graduated from the University of Tübingen with a doctorate in medicine. In 1588 Beyer returned to his native Frankfurt and began working as a physician; a year later he was appointed Physicus ordinarius - his duties included overseeing the city's health care and pharmacy system.
In 1614 Beyer took up the position of senior burgomaster of Frankfurt, but during the Fetmilch Rebellion he became involved in conflict, was forced to resign and returned to science.
He had the richest library of scientific books, numbering about 2500 volumes, wrote scientific works on astronomy and mathematics, engaged in medical activity, having invented the famous Frankfurt pills. Beyer carried on a lively correspondence with scientists, including mathematician Johannes Kepler, dealing with decimal fractions. Beyer bequeathed his rich inheritance to the city and to charity.


Joseph Georg Böhm was an Austrian astronomer, astrophysicist, cartographer, mathematician, and educator.
At the University of Prague, Böhm attended lectures in mathematics, physics, astronomy, and, after receiving his doctorate, became an assistant at the Vienna Observatory under Joseph Johann von Littrow. He then worked at the observatory at Othen and taught mathematics at the University of Salzburg. In 1839 he was appointed professor of mathematics and practical geometry at the University of Innsbruck, and in 1848 he was elected its rector. In 1852 Böhm was appointed director of the Prague Observatory and professor of theoretical and practical astronomy at the University of Prague.
Georg Böhm published several significant astronomical papers on solar observation. He is the creator of several instruments for astronomical measurements and observations, and he designed the Uranoscope and Universal Gnomon for amateur astronomical observations for the general public. As a member of the commission for the repair of the Prague Astronomical Clock, which he joined in 1865, he wrote a detailed description of it in the work Beschreibung der alterthümlichen Prager Rathausuhr. In addition to astronomy and its popularization, he was also involved in agriculture, economics, and geodesy. One of his important works is Ballistic Experiments (1865).


Bernard Bolzano, full name Bernard Placidus Johann Nepomuk Bolzano, was an Italian-born Czech scientist, mathematician, logician, philosopher and theologian.
Bolzano graduated from the University of Prague and was immediately appointed professor of philosophy and religion at the university. Within a few years, however, Bolzano had already shown himself to be a free thinker with his teachings on the social costs of militarism and the needlessness of war. He called for a complete reform of the educational, social, and economic systems that would direct the nation's interests toward peace rather than armed conflict between states. In 1819, Bolzano was expelled from the university for his beliefs and thereafter turned his full attention to works on social, religious, philosophical, and mathematical issues.
Bolzano held advanced views on logic, mathematical quantities, limits, and continuity. He is the author of the first rigorous theory of real numbers and one of the founders of set theory. In his studies of the physical aspects of force, space, and time, he proposed theories opposed to those advanced by the German philosopher Immanuel Kant. His contributions to logic, in particular, established his reputation as the greatest logician of his time. Much of his work remained unpublished during his lifetime and was not widely disseminated until the late nineteenth and early twentieth centuries, when a number of his conclusions were reached independently.
Bolzano was multi-talented in various fields of science to which he made significant contributions. His published works include The Binomial Theorem (1816), A Purely Analytic Proof (1817), The Functional Model and the Scientific Model (1834), An Attempt at a New Statement of Logic (1837), and The Paradoxes of Infinity (1851).
Among other things, Bolzano was also a great philanthropist. Together with his friends and students, he supported the activities of almshouses, homes for the blind, loan banks for the working class, libraries, and elementary schools in rural areas.


Giovanni Alfonso Borelli was an Italian universalist scientist of the 17th century Scientific Revolution, the founder of biomechanics.
He studied mathematics under Benedetto Castelli (1577-1644) in Rome. In the 1640s Borelli was appointed to the chair of mathematics at the University of Messina and at Pisa in 1656. After 12 years at Pisa and numerous disputes with colleagues, Borelli left the university. In 1667 Borelli returned to the University of Messina, where he engaged in literary and historical studies, studied the eruption of the volcano Etna, and continued to work on the problem of muscular movement of animals and other bodily functions according to the laws of statics and dynamics. In 1674 he was accused of participating in a conspiracy to liberate Sicily from Spain and fled to Rome.
Borelli is known primarily for his attempts to explain muscular movement and other bodily functions according to the laws of statics and dynamics. His best-known work is De Motu Animalium (1680-81; "On the Motion of Animals"). Borelli calculated the forces required for balance in the various joints of the human body, long before Newton published his Laws of Motion. Borelli was the first to realize that musculoskeletal levers increase motion, not force, so muscles must produce much greater forces than those that resist motion. He was also one of the first microscopists: he made microscopic studies of blood circulation, nematodes, textile fibers, and spider eggs. Borelli also authored works on physics, medicine, astronomy, geology, mathematics, and mechanics.


Ismaël Boulliau (Boulliaud), also known as Ismael Bullialdus, was a French astronomer and mathematician who followed the teachings of Copernicus.
Boulliau worked as a librarian for many years and had the opportunity to study the scientific works of Copernicus, Galileo, and Kepler, and as a result became a strong supporter of the heliocentric system of the world. Boulliau was also intimately acquainted with Huygens, Gassendi, Pascal and other prominent scientists of the time, and he translated many works from Greek into Latin.
Boulliau's main astronomical work, published in 1645, was Astronomia philolaica (Astronomy of Philolaus, named after the ancient Greek Pythagorean philosopher Philolaus, who promoted the idea of the motion of the Earth). In it, he supported Kepler's first law that the planets move on ellipses, and provided new evidence for this. Isaac Newton, in Book III of The Mathematical Beginnings of Natural Philosophy, relies on measurements of the magnitudes of planetary orbits determined from observations by Kepler and Boulliau.
Boulliau was also interested in history, theology, classical studies, and philology. He was active in the Republic of Letters, an intellectual community whose members exchanged ideas.


Joachim Bouvet was a French Jesuit monk and missionary who worked in China.
Joachim Bouvet was one of six Jesuit mathematicians chosen by Louis XIV to travel to China as his envoys and work as missionaries and scholars. In 1687 in Beijing, Bouvet began this work, especially in mathematics and astronomy, and in 1697 the Chinese emperor Kangxi (1654-1722) sent him as ambassador to the French king. Kangxi expressed his wish that Bouvet should bring more missionary scientists with him. Thus, in addition to his scholarly work, Bouvet was also an accomplished diplomat and served as a liaison between the Chinese Emperor Kangxi and King Louis XIV of France.
Bouvet brought to France a manuscript describing Kangxi's life with an eye for diplomatic subtleties, as well as a collection of drawings depicting graceful Chinese figures in traditional and ceremonial dress. The first French edition of The Historical Portrait of the Emperor of China was published in Paris in 1697, and was subsequently translated and published in other languages. And Bouvet returned to China in 1699 with ten new missionaries and a collection of King Louis XIV's engravings for Emperor Kangxi. He remained in China for the rest of his life.


Tycho Brahe, born Tyge Ottesen Brahe, more commonly called Tycho, was a prominent Danish astronomer, astrologer, and alchemist of the Renaissance.
As a young man he traveled extensively throughout Europe, studying in Wittenberg, Rostock, Basel, and Augsburg and acquiring mathematical and astronomical instruments. In 1572 Tycho unexpectedly even for himself discovered a new star in Cassiopeia, and the publication of this turned the young Dane into an astronomer of European reputation. For further astronomical research he established an observatory and gathered around him modern progressive scientists.
Besides practicing astronomy, Tycho was an artist, scientist, and craftsman, and everything he undertook or surrounded himself with had to be innovative and beautiful. He even founded a printing house to produce and bind his manuscripts in his own way, and he perfected sanitary ware for convenience. His development of astronomical instruments and his work in measuring and fixing the positions of the stars laid a solid foundation for future discoveries.
Tycho's observations - the most accurate possible before the invention of the telescope - included a comprehensive study of the solar system and the precise positions of more than 777 fixed stars. What Tycho accomplished using only his simple instruments and intellect remains a remarkable achievement of the Renaissance.
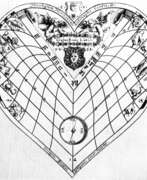

Georg Brentel the Younger was a German draftsman, engraver, and author of works on sundials and instrumentation.
He was the son of the cartographer Hans Brentel (1532-1614) and nephew of the armorial artist Georg Brentel the Elder (1525-1610). He always showed an interest in mathematics and astronomy, writing papers on these subjects and making instruments.
Brentel was particularly fond of designing sundials, and wrote several instructions for assembling various types of sundials - round and cubic, cross-shaped and heart-shaped.


Victor Yakovlevich Bunyakovsky (Russian: Ви́ктор Я́ковлевич Буняко́вский) was a Russian mathematician, teacher, historian of mathematics, vice-president of the Academy of Sciences in 1864-1889. He made a significant contribution to number theory.


Augustin Louis Cauchy was a French mathematician and mechanic, military engineer, and founder of mathematical analysis.
Cauchy studied at the École Polytechnique and at the Paris School of Bridges and Roads. After becoming a military engineer, he went to Cherbourg in 1810 to work on harbors and fortifications for Napoleon's English invasion. Here he began independent mathematical research. Cauchy returned to Paris in 1813, and Lagrange and Laplace convinced him to devote himself entirely to mathematics. The following year he published a memoir on definite integrals, which formed the basis of the theory of complex functions. From 1816, Cauchy held professorships at the Faculty of Natural Sciences, the Collège de France, and the École Polytechnique in Paris.
Cauchy developed the foundation of mathematical analysis, and made enormous contributions to analysis, algebra, mathematical physics, and many other areas of mathematics. He almost single-handedly founded the theory of functions of a complex variable, which has extensive applications in physics. Cauchy's greatest contributions to mathematics are published primarily in three of his treatises, "Courses in Analysis at the Royal Polytechnic School" (1821), "Summary of Lessons on Infinitesimal Calculus" (1823), and "Lessons on the Application of Infinitesimal Values in Geometry" (1826-28). In all, Augustin Louis Cauchy wrote about 800 scientific articles.
Cauchy was a member of the Paris Academy of Sciences, the Royal Society of London, the St. Petersburg Academy of Sciences, and other academies.


Bonaventura Francesco Cavalieri (Latin: Bonaventura Cavalerius) was an Italian mathematician and a Jesuate. He is known for his work on the problems of optics and motion, work on indivisibles, the precursors of infinitesimal calculus, and the introduction of logarithms to Italy. Cavalieri's principle in geometry partially anticipated integral calculus.


Nicolas Chuquet was a 15th century French mathematician.
The exact dates of birth and death of this scientist are not known, nor are the places of birth. Chuquet received a Bachelor of Medicine degree from the University of Paris, went to Italy in the early 1470s, and around 1480 moved to Lyon, where he worked as a physician, mathematics teacher, and scribe. He is also known to have translated Latin works into French.
In 1484 he wrote his major algebraic work, the treatise Le Triparty en la Science des Nombres (The Science of Numbers in Three Parts), now considered one of the most original mathematical texts of the 15th century. At the time, arithmeticians lacked even the most basic notations for addition subtraction, multiplication, and division. Chuquet was one of the first to propose these symbols; he also introduced the names of large numbers into common use: billion, trillion, etc. In addition to general arithmetic and rules for calculating roots, the treatise contains a doctrine of equations and a collection of problems.
This treatise was published only in 1880, but the works of Nicolas Chuquet had a significant influence on the development of algebra, and they were consistently supplemented and expanded by scientists of the following generations.


Nicolaus Copernicus (Polish: Mikołaj Kopernik) was a Polish and German scientist, astronomer, mathematician, mechanic, economist, and Renaissance canonist. He was the author of the heliocentric system of the world, which initiated the first scientific revolution.
Copernicus studied the humanities, including astronomy and astrology, at the University of Krakow and at the University of Bologna in Italy. Together with other astronomers, including Domenico Maria de Novara (1454-1504), he was engaged in observing the stars and planets, recording their movements and eclipses. At the time, medicine was closely related to astrology, as the stars were believed to influence the human body, and Copernicus also studied medicine at the University of Padua between 1501 and 1503.
Nicolaus Copernicus, based on his knowledge and observations, was the first to suggest that the Earth is a planet that not only revolves around the sun every year, but also rotates once a day on its axis. This was in the early 16th century when people believed the Earth to be the center of the universe. The scientist also suggested that the Earth's rotation explained the rising and setting of the Sun, the movement of the stars, and that the cycle of the seasons was caused by the Earth's rotation around itself. Finally, he correctly concluded that the Earth's motion in space causes the planets to move backwards across the night sky, the so-called retrograde direction.
Although Copernicus' model was not completely correct, it laid a solid foundation for future scientists, such as Galileo, who developed and improved mankind's understanding of the motion of celestial bodies. Copernicus completed the first manuscript of his book De Revolutionibus Orbium Coelestium (On the Rotation of the Celestial Spheres) in 1532. In it, the astronomer outlined his model of the solar system and the paths of the planets. However, he published the book only in 1543, just two months before his death, and dedicated it to Pope Paul III. Perhaps for this reason, and also because the subject matter was too difficult to understand, but the church did not finally ban the book until 1616.


Estienne de la Roche, also known as Estienne de Villefranche, was a French mathematician.
He is known for having taught mathematics in Lyon for 25 years as a professor of mathematics. In 1520 he published the Arismatics, considered at the time the best reference book on algebra.


René Descartes was a French philosopher, mathematician, and natural scientist who is considered the founder of modern philosophy.
Descartes was a very versatile scientist: besides numerous philosophical reflections, he wrote works on optics, meteorology and geometry. Contemporaries noted his extensive knowledge in many sciences. Descartes owns the famous saying "I think, therefore I exist" (best known in the Latin formulation "Cogito, ergo sum", although it was originally written in French: "Je pense, donc je suis").
He developed a metaphysical dualism that radically distinguished between mind, whose essence is thought, and matter, whose essence is extension in three dimensions. Descartes' metaphysics is rationalistic, based on the postulation of innate ideas of mind, matter, and God, but his physics and physiology, based on sense experience, are mechanistic and empirical.
Unlike his scientific predecessors, who felt a holy awe at the incomprehensibility of the divine essence of the universe, Descartes admired the ability of the human mind to understand the cosmos and to generate happiness itself, and rejected the view that human beings were inherently unhappy and sinful. He believed that it was inappropriate to pray to God to change the state of things and the world; it was much more productive to change oneself.


Lewis Carroll, real name Charles Lutwidge Dodgson, was a British writer and photographer, philosopher and logician, and professor of mathematics.
In 1851 Lewis entered one of the best colleges in Oxford - Christ Church. Showing an extraordinary aptitude for mathematics, soon he was able to give lectures himself, and for the next quarter of a century he was a professor of mathematics at Oxford. In parallel with his studies, the young man began to compose short stories and poems, publishing them under a pseudonym.
And then he wrote the famous "Alice's Adventures in Wonderland" (Alice's Adventures in Wonderland, 1865) and "Alice in Looking-Glass" (Through the Looking-Glass, and What Alice Found There, 1871). These books quickly became popular, they were translated into numerous languages, and then repeatedly screened. The prototype of the main character was four-year-old Alice Liddell, the daughter of the new dean of the college where Carroll taught. Lewis Carroll also wrote "The Knotty Story", a humorous poem "Hunting the Snark", "Mathematical Curiosities", "Sylvia and Bruno" and other books. Carroll himself considered his main work a slightly absurd novel-tale "Sylvia and Bruno" (1889-1893).
Under his real name, the writer-mathematician published scientific works on mathematics and logic, he also owns a number of popular books on entertaining mathematics. Lewis Carroll left Oxford only once - in 1867, visiting Russia as part of a delegation of the Anglican Church on the route St. Petersburg-Moscow-Nizhny Novgorod. This was Carroll's only overseas trip, and he described it in his Diary of a Trip to Russia 1867. Lewis Carroll was also a talented chess player and amateur inventor. Photography was also a big part of the writer's life.


Johann Dryander, born Johann Eichmann, was a German medical anatomist, mathematician and astrologer.
He studied anatomy and medicine at the University of Paris and the University of Erfurt, and in 1535 became professor of medicine at the University of Marburg. A year later, Dryander performed two public autopsies, making the first illustrated description of the dissection of the human brain. Dryander titled his book Anatomiae, hoc est, corporis humani dissectionis pars prior ("Anatomy, that is, the dissection of the human body, part one," suggesting a sequel, which, however, did not follow.
His work made a significant contribution to the development of modern anatomy. Toward the end of his life, Dryander also dabbled in astrology and mathematics.


Euclid (Greek: Εὐκλείδης) was an ancient Greek mathematician active as a geometer and logician. Considered the "father of geometry", he is chiefly known for the Elements treatise, which established the foundations of geometry that largely dominated the field until the early 19th century. His system, now referred to as Euclidean geometry, involved new innovations in combination with a synthesis of theories from earlier Greek mathematicians, including Eudoxus of Cnidus, Hippocrates of Chios, Thales and Theaetetus. With Archimedes and Apollonius of Perga, Euclid is generally considered among the greatest mathematicians of antiquity, and one of the most influential in the history of mathematics.


Leonhard Euler was the greatest mathematician of the 18th century and history in general.
Euler brilliantly graduated from the University of Basel and entered the St. Petersburg Academy of Sciences, then began to work at the Berlin Academy, and later to lead it. In 1766, the scientist received an invitation from the Russian Empress Catherine II and again came to St. Petersburg to continue his scientific work.
Here he published about 470 works in a wide variety of fields. One of them is a large-scale work "Mechanics" - an in-depth study of this science, including celestial mechanics. Euler by that time was practically blind, but continued to be actively engaged in science, in the records he was helped by his son Johann Albrecht and stenographers. Leonhard Euler made many fundamental discoveries that brought great benefit to mankind.
His massive contribution to the development of mathematics, mechanics, physics and astronomy cannot be overestimated, and his knowledge in the most diverse branches of science is admirable. During his lifetime, he published more than 850 works that contain in-depth studies of botany, chemistry, medicine, ancient languages, and music. Euler held membership in many academies of science around the world.


Jacobus Faber Stapulensis or Jacques Lefèvre d'Étaples was a French humanist, Catholic theologian, philosopher, music theorist and mathematician. He taught at the University of Paris. He is best known as the first translator into French of the complete text of the Bible.


Johannes Faulhaber was a German mathematician and fortification engineer.
He was a weaver, but studied mathematics and showed such aptitude that the city authorities appointed him the city mathematician and surveyor, a surveyor. In 1600, Faulhaber opened his own school in Ulm, and worked on the fortification of Basel, Frankfurt, and many other cities. He also designed water wheels in Ulm and made mathematical and geodetic instruments, particularly for military purposes.
Among the scientists with whom Faulhaber collaborated were Kepler and van Ceulen. He made the first German publication of Briggs' logarithms, and also made the first illustrated descriptions of Galileo's compass.


Joseph Fourier, full name Jean-Baptiste Joseph Fourier, was a French mathematician and physicist and historical Egyptologist.
Fourier famously accompanied Napoleon Bonaparte on his Egyptian expedition in 1798 as a scientific advisor and was appointed secretary of the Institute of Egypt. During the occupation of Egypt, Fourier worked in the French administration, supervised archaeological excavations, and worked to shape the educational system.
But the main thing in Fourier's life was science. Back in France, he studied the mathematical theory of heat conduction, established the partial differential equation governing heat diffusion, and solved it using an infinite series of trigonometric functions. Fourier showed that heat diffusion obeyed simple observable physical constants that could be expressed mathematically. His work The Analytic Theory of Heat (1822) had a great influence on the development of physics and pure mathematics.
Joseph Fourier was a member of the Paris Academy of Sciences, the French Academy, a foreign honorary member of the St. Petersburg Academy of Sciences, and a member of the Royal Society of London.


Gemma Frisius, born Jemme Reinerszoon Frisius, was a Dutch mathematician, physician, cartographer, philosopher, engraver, and master of astronomical instruments.
He taught mathematics and medicine at the University of Leuven and applied his mathematical knowledge to astronomy, geography, and map-making. Frisius participated in the creation of the latest globes and used mathematics in geodesy and navigation in new ways and invented or improved many instruments, including the cross staff, the astrolabe, and the astronomical rings (also known as "Gemma rings"). He ran a workshop for making such instruments.
Frisius is credited with being one of the founders of the Dutch school of cartography.


Georg Galgemair was a German mathematician and astrologer.
He was born into the family of the burgomaster of Donauwörth, was a pupil of Philip Apian, and then a master of mathematics at the University of Tübingen in 1585. After completing his studies, Galgemair began teaching at Lauingen in 1588.
His work on proportional circles led to the development of gnomonics. In the history of science, Galgemair is known for his works on mathematical instruments. As a calendar maker, he succeeded in 1606 in obtaining an imperial privilege for his calendars.


Galileo Galilei was an Italian naturalist, physicist, mechanic, astronomer, philosopher, and mathematician.
Using his own improved telescopes, Galileo Galilei observed the movements of the Moon, Earth's satellites, and the stars, making several breakthrough discoveries in astronomy. He was the first to see craters on the Moon, discovered sunspots and the rings of Saturn, and traced the phases of Venus. Galileo was a consistent and convinced supporter of the teachings of Copernicus and the heliocentric system of the world, for which he was subjected to the trial of the Inquisition.
Galileo is considered the founder of experimental and theoretical physics. He is also one of the founders of the principle of relativity in classical mechanics. Overall, the scientist had such a significant impact on the science of his time that he cannot be overemphasized.


Pierre Gassendi was a French Catholic priest, Epicurean philosopher, mathematician, astronomer and researcher of ancient texts.
He taught rhetoric at Diné and philosophy at Aix-en-Provence. Gassendi's Syntagma philosophicum, the result of his historical research and philosophical reflections, is a well-known work. Several of his works on astronomy, physics and mechanics were also published in the 17th century.


Sir John Frederick William Herschel, 1st Baronet was a British astronomer and son of the Uranus discoverer Wilhelm Herschel. He is credited with the first double star and nebula catalogues of the southern starry sky, which he observed during a five-year stay near Cape Town.


Christiaan Huygens van Zeelhem was a Dutch mechanic, physicist, mathematician, inventor and astronomer who formulated the wave theory of light.
An admirer of Descartes, Huygens preferred to conduct new experiments himself to observe and formulate laws. In physics, he contributed to the development of the crucial Huygens-Fresnel principle, which applies to wave propagation. He also extensively investigated free fall. He experimentally proved the law of conservation of momentum. He derived the law of centrifugal force for uniform circular motion.
He also invented the pendulum clock, discovered centrifugal force and the true shape of Saturn's rings as well as its moon Titan. Huygens is considered the first theoretical physicist to use formulas in physics and one of the founders of theoretical mechanics and probability theory.


Johannes Kepler was a German mathematician and astronomer who discovered that the Earth and planets move around the Sun in elliptical orbits.
Kepler created the three fundamental laws of planetary motion. He also did seminal work in optics and geometry, calculated the most accurate astronomical tables, and made many inventions and discoveries in physics on which further scientific discoveries by advanced scientists were based.


Athanasius Kircher was a German scholar, inventor, professor of mathematics and oriental studies, and a friar of the Jesuit order.
Kircher knew Greek and Hebrew, did scientific and humanities research in Germany, and was ordained in Mainz in 1628. During the Thirty Years' War he was forced to flee to Rome, where he remained for most of his life, serving as a kind of intellectual and information center for cultural and scientific information drawn not only from European sources but also from an extensive network of Jesuit missionaries. He was particularly interested in ancient Egypt and attempted to decipher hieroglyphics and other riddles. Kircher also compiled A Description of the Chinese Empire (1667), which was long one of the most influential books that shaped the European view of China.
A renowned polymath, Kircher conducted scholarly research in a variety of disciplines, including geography, astronomy, mathematics, languages, medicine, and music. He wrote some 44 books, and more than 2,000 of his manuscripts and letters have survived. He also assembled one of the first natural history collections.


Gottfried Wilhelm Leibniz was a German philosopher and a prominent polymath in many fields of science.
Leibniz was a universal genius; he showed his talents in logic, mathematics, mechanics, physics, law, history, diplomacy, and linguistics, and in each of the disciplines he has serious scientific achievements. As a philosopher, he was a leading exponent of 17th-century rationalism and idealism.
Leibniz was a tireless worker and the greatest scholar of his time. In the fate of Leibniz, among other things, there is one interesting page: in 1697, he accidentally met the Russian Tsar Peter I during his trip to Europe. Their further meetings led to the realization of several grandiose projects in Russia, one of which was the establishment of the Academy of Sciences in St. Petersburg.
Gottfried Wilhelm Leibniz was also the founder and first president of the Berlin Academy of Sciences and a member of the Royal Society of London.


James Clerk Maxwell was a British physicist, mathematician and mechanic, and a Fellow of the Royal Society of London.
James Clerk Maxwell was one of the most influential scientists of the nineteenth century. His theoretical work on electromagnetism and light largely determined the direction physics would take in the early 20th century. Maxwell conducted research in a number of areas, but was particularly preoccupied with the nature of Saturn's rings. In 1860 he obtained a professorship at King's College, London, and it was his years there that were most fruitful. For his electromagnetic theory,
Maxwell is most often credited with fundamentally changing the course of physics. Maxwell saw electricity not just as another branch of physics, but "as an aid to the interpretation of nature." He showed the importance of electricity to physics as a whole by advancing "the important hypothesis that light and electricity are the same in their ultimate nature." This theory, one of the most important discoveries in nineteenth-century physics, was Maxwell's greatest achievement and laid the foundation for Einstein's theory of relativity.


Marin Mersenne (also Marinus Mersennus or le Père Mersenne) was a French mathematician, physicist, philosopher and theologian, and music theorist.
Mersenne was educated at the Jesuit college of La Fleche and went on to study theology in Paris. He also became a member of the Order of the Minims and taught philosophy and theology at Nevers. He developed his ideas about the essence of the world and knowledge, insisting on the importance of experimentation and observation, and contrasted the rational natural world with human reason.
Beginning in 1635, Mersenne founded the Académie Parisienne, the forerunner of the French Academy of Sciences, where France's leading mathematicians and natural philosophers gathered. It provided a forum for the exchange of ideas among scientists and promoted the works of René Descartes and Galileo. The scientist's most famous achievement in mathematics was finding a formula for generating prime numbers, known today as Mersenne's Numbers. In 1644, Mersenne published his studies of Mersenne numbers and their relationship to prime numbers. His work in number theory and arithmetic proved pivotal to the development of mathematics in the seventeenth century.
He corresponded with many other scientists of the era, such as René Descartes, Blaise Pascal, and Pierre Fermat. However, his contributions extend much further, through his role in disseminating the work of the outstanding minds of his time. Mersenne traveled extensively throughout Europe, bringing new scientific ideas to France. He was an important mediator in the exchange of knowledge and contributed to the advancement of science in his era.


Isaac Newton was an English mathematician, physicist, astronomer, alchemist, theologian, and author (described in his time as a "natural philosopher"), widely recognised as one of the greatest mathematicians and physicists and among the most influential scientists of all time. He was a key figure in the philosophical revolution known as the Enlightenment. His book Philosophiæ Naturalis Principia Mathematica (Mathematical Principles of Natural Philosophy), first published in 1687, established classical mechanics. Newton also made seminal contributions to optics, and shares credit with German mathematician Gottfried Wilhelm Leibniz for developing infinitesimal calculus.
In the Principia, Newton formulated the laws of motion and universal gravitation that formed the dominant scientific viewpoint until it was superseded by the theory of relativity. Newton used his mathematical description of gravity to derive Kepler's laws of planetary motion, account for tides, the trajectories of comets, the precession of the equinoxes and other phenomena, eradicating doubt about the Solar System's heliocentricity. He demonstrated that the motion of objects on Earth and celestial bodies could be accounted for by the same principles. Newton's inference that the Earth is an oblate spheroid was later confirmed by the geodetic measurements of Maupertuis, La Condamine, and others, convincing most European scientists of the superiority of Newtonian mechanics over earlier systems.


Giovanni Vincenzo Petrini was an Italian priest and theologian, philosopher, mathematician, and expert in mineralogy.
Along with Scipio Breislacus, Petrini was one of the founders of Italian volcanology. He taught philosophy and mathematics, theology, but specialized in mineralogy and created the Mineralogical Cabinet in Nazareth. This museum was famous in Europe and was visited, among others, by Emperor Joseph II, who gave him rare specimens from the lands of the Empire and especially from Hungary.
Giovanni Petrini was the author of the catalog Gabinetto mineralogico del Collegio Nazareno ("The Mineralogical Cabinet of the Nazarene Collegium, described by external features and distributed by component parts" (Rome, 1791-1792). The specimens in it are classified according to a standard structure: salts, earths, bitumens, combustibles, and metals. There is also a section on gemstones.


Giuseppe Piazzi was an Italian astronomer, mathematician and priest.
Around 1764 Piazzi became a Theatine priest, in 1779 he was appointed professor of theology in Rome, and in 1780 - professor of higher mathematics at the Academy of Palermo. Later, with the assistance of the Viceroy of Sicily, he founded an observatory in Palermo. There he compiled his great catalog of the positions of 7,646 stars and showed that most stars move relative to the Sun. There, on January 1, 1801, Piazzi also discovered the asteroid Ceres.
Giuseppe Piazzi's merits were appreciated: he was a member of the Royal Society of London, a foreign honorary member of the St. Petersburg Academy of Sciences and a foreign member of the Paris Academy of Sciences. A crater on the Moon is named in his honor.


Agostino Ramelli was an Italian military engineer and mechanic who worked in the fields of fortification and practical mechanics.
Ramelli studied mathematics, mechanics, and engineering under Giacomo di Marignano, who is considered a disciple of Leonardo da Vinci. He first showed his talents as a mechanic during Louis XIII's military campaign by constructing a mine under a bastion.
Ramelli invented many mechanisms that impressed his contemporaries, including their special aesthetic appeal. His most popular creation is the so-called Ramelli Book Wheel, a rotating reading table. Agostino Ramelli positioned his invention as a sleek design that allowed access to several books without having to get up from his seat.
Ramelli wrote and illustrated a book of engineering projects, Le various et artificiose machine ("Various and Artificial Machines"). The book contains 195 designs, over 100 of which are water-lifting machines, such as water pumps or wells, as well as bridges, mills, and so on. This very interesting book for our contemporaries is still published and is still in demand.


Regiomontanus, real name Johannes Müller, was a 15th-century German astronomer and mathematician, one of the first printers.
The son of a miller, he entered the University of Leipzig at the age of 11 and later transferred to the University of Vienna. In 1452, Regiomontanus earned a bachelor's degree and then a master's degree. With his teacher, the mathematical astronomer Georg von Peyerbach (d. 1461), he spent the next years practicing astronomy and astrology, including observations of eclipses and comets, making astronomical instruments, and compiling horoscopes for the court of Holy Roman Emperor Frederick III.
Regiomontanus was also seriously involved in mathematics, publishing his major work on trigonometry, On All Kinds of Triangles (1462-1464). From 1467 to 1471, Regiomontanus lived in Hungary as astrologer to Hungarian King Matyas I and Archbishop Janos Vitez. Then in Nuremberg, Germany, he opened an instrument workshop, established a printing house, and continued his planetary observations. The scholar planned to print extensive publications on classical, medieval, and modern mathematical sciences, but not all plans came to fruition.


Vincenzo Renieri, born Giovanni Paolo, was an Italian priest, astronomer and mathematician.
Renieri was a member of the Olivetan Order and traveled throughout Italy. In 1633 in Siena, he met the already blind Galileo, who, appreciating his knowledge, instructed him to update his astronomical tables of the motion of the satellites of Jupiter, adding new ones. Rainieri later met the astronomer and scientist Vincenzo Viviani (1622-1703), with whom he worked for many years, continuing Galileo's observations of Jupiter's moons.
Renieri was also professor of mathematics at the University of Pisa and taught Greek there. In 1639 he published his work Tabulae Mediceae secundorum Mobilium Universales in Florence. One of the lunar craters is named after Renieri.


Paolo Ricci (Italian: Paolo Ricci, Latin: Paulus Ricius, German: Paul Ritz), also known as Ritz, Riccio, or Paulus Israelita, was a humanist convert from Judaism, a writer-theologian, Kabbalist, and physician.
After his baptism in 1505 he published his first work, Sol Federis, in which he affirmed his new faith and sought through Kabbalah to refute modern Judaism. In 1506 he moved to Pavia, Italy, where he became a lecturer in philosophy and medicine at the university and met Erasmus of Rotterdam. Ricci was also a learned astrologer, a professor of Hebrew, philosophy, theology, and Kabbalah, a profound connoisseur and translator of sacred texts into Latin and Hebrew, and the author of philosophical and theological works.
Paolo Ricci was a very prolific writer. His Latin translations, especially the translation of the Kabalistic work Shaare Orach, formed the basis of the Christian Kabbalah of the early 16th century.


Franz Xaver Freiherr (from 1801) von Zach, Baron (Hungarian: Zách János Ferenc) was an Austro-German astronomer, surveyor, mathematician, science historian and officer of Hungarian origin. He rendered outstanding services in the exploration of the solar system and the organization of international astronomy, after whom a lunar crater (Zach) and an asteroid ((999) Zachia) were named, among others. He was also the founder of the first scientific journals and organised the first astronomical congress in 1798.


Johann Zahn (German: Johann or Johannes Zahn) was a German scientist and philosopher, optician and astronomer, mathematician and inventor.
Zahn studied mathematics and physics at the University of Würzburg, was professor of mathematics at the University of Würzburg, and served as a canon of the Order of Regular Canon Premonstratensians. His other activities were optics as well as astronomical observations.
In 1686 Johann Zahn invented and designed a portable camera obscura with fixed lenses and an adjustable mirror, which is the prototype of the camera. In his treatise on optics, Oculus Artificialis Teledioptricus (1702), Zahn gives a complete picture of the state of optical science of his time. He begins with basic information about the eye and then moves on to optical instruments. The book is aimed at eighteenth-century microscope and telescope enthusiasts and includes all the necessary details of construction, from lens grinding to drawings.