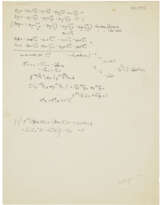ID 1249825
Lot 79 | Albert Einstein (1879-1955)
Valeur estimée
£ 40 000 – 60 000
Autograph letter signed (with initials, 'A.E.') to Melanie Serbu, Huntington, Long Island, 15 July 1937
In German. Two pages, 280 x 2150mm, ten mathematical formulae (including Newton’s second law of motion) within the text.
'On the concept of liquids as quasi-solids': Einstein mentoring a young female scientist, renewing themes from his own doctoral thesis of 1905. Einstein recommends a course of research for Serbu’s doctoral dissertation, on testing viscosity theory by modelling the deformation of liquids, and sketches out the mathematical formulas for a theoretical treatment.
'I am glad that you agree with me on the concept of liquids as quasi-solids. Do not talk too much about this matter with others; I actually think that this could later make a nice topic for your doctoral thesis, and I am thinking primarily of the theoretical implementation of the idea. It probably won't be entirely new. For example, in gas theory the term "relaxation time" (Boltzmann) is common and has something to do with this. / I will outline the matter for you as regards pure shear deformations ...'. Einstein goes on to express the question in scientific terms, defining terms for shear force and elongation, and setting out an equation for their relationship in an ideal solid body, and thence in a fluid, introducing terms for increasing deformation or relaxation to produce a first equation, and then providing a second 'equation of motion (mass.acceleration= force)': the two equations together 'determine the movement ... But what about viscosity?'. Einstein then derives a viscosity coefficient from equation I, and shows how the relaxation rate can be derived from this: 'You can now amuse yourself by integrating Equations I and II for temporal sinusoidal waves. One can thus predict how high the frequencies need to be in order for the behaviour of the liquid in relation to waves to be noticeably different from the predictions of the usual viscosity theory. According to this, the left side in [equation] I may be neglected in comparison with the second term on the right side'; he suggests an equation which can replace equation l, and concludes 'One has in fact all the transitions between a solid body and an ideal viscous fluid'.
The present letter's theme of testing viscosity theory is immediately reminiscent of Einstein’s doctoral thesis, 'A New Determination of Molecular Dimensions' (1905), a work which had incalculable implications for the development of 20th century science both practically and methodologically, and remains Einstein’s most cited work. By working statistically with the viscosity factors of liquids, Einstein's thesis derives a new theoretical method for calculating the size of atoms and molecules. Einstein himself immediately applied his findings and methodology to the problem of Brownian motion, thereby proving both the actual physical existence of atoms and the validity of the molecular-kinetic theory of thermodynamics. Einstein was in fact a pioneer in the field of statistical mechanics, developing his own distinctively elegant and 'economical' methodology in the 1902-04 period and immediately applying it to real physical problems in 1905 (including his Nobel- Prize winning work with the photon). When Jean Perrin empirically confirmed the existence of atoms in 1908 (for which he won the Nobel Prize in 1926), Einstein’s statistical approach to problem-solving became the methodological paradigm for 20th century physics (including probabilistic quantum mechanics).
Here, 32 years later, he returns to the question of viscosity, prompted by his correspondence with the young Romanian mathematician Melanie Serbu (1909-1979): in May 1937, she had quizzed Einstein on her view of the differing states of matter (solid, liquid, gas), and the potential for viewing them as on a continuum of differing degrees of viscosity. In a letter of 25 May 1937, Einstein initially disagreed with her characterisation of solid bodies, but put forward his own view that liquids may be considered as solid bodies with low resistance to deformation. The present letter goes a step further in testing the prevailing theory of viscosity by examining 'all the transitions between a solid body and an ideal viscous fluid'. Serbu did in fact go on to work on viscosity in her dissertation, although not on the lines sketched by Einstein here. Her correspondence with Einstein had begun as early as 1928, when she was only 19; in the post-war years she went on to work as a mathematician at the university of Braşov, before emigrating to Israel in 1958.
Given Einstein’s own desire for secrecy in the matter of this letter, one can only speculate what physical application Einstein might have had in mind with the theoretical analysis he is here proposing. Einstein did considerable work with molecular physics over course of his career – ranging from Bose-Einstein condensates to mass-energy ratios in ideal gas – and it is entirely possible that he is here contemplating further work with quantum-mechanical phases of matter. This letter has not been formally published, and it appears to be unstudied by historians of science.
| Artiste: | Albert Einstein (1879 - 1955) |
|---|---|
| Lieu d'origine: | Europe de l'Ouest, Allemagne, Europe, Suisse |
| Catégorie maison de vente aux enchères: | Lettres, documents et manuscrits, Médecine et sciences, Livres et manuscrits |
| Artiste: | Albert Einstein (1879 - 1955) |
|---|---|
| Lieu d'origine: | Europe de l'Ouest, Allemagne, Europe, Suisse |
| Catégorie maison de vente aux enchères: | Lettres, documents et manuscrits, Médecine et sciences, Livres et manuscrits |
| Adresse de l'enchère |
CHRISTIE'S 8 King Street, St. James's SW1Y 6QT London Royaume-Uni | |
|---|---|---|
| Aperçu |
| |
| Téléphone | +44 (0)20 7839 9060 | |
| Commission | see on Website | |
| Conditions d'utilisation | Conditions d'utilisation |
Plus du Créateur
Termes connexes
Déposer un ordre
Déposer un ordre par téléphone Ordre
| Numéro de lot | 79 |
|---|---|
| Maison de vente aux enchères | CHRISTIE'S |
| Date de l'enchère | 10.07.2024 |
Votre ordre: £ 40 000
Téléphones pour la communication:
+34827387, +232346832
C'est votre offre maximale qui est transmise à la maison des ventes aux enchères. Si l'offre maximale est plus en haut que le prix limite de l'article, le commissaire-priseur offrira pour vous jusqu'à la hauteur de l'offre maximale.
C'est votre enchère maximale, qui est transmise à la maison de vente aux enchères. Si l'enchère maximale est plus haut que le prix minimal de l'article, le commissaire-priseur offrira pour vous jusqu'à la hauteur de l'enchère maximale.
| Numéro de lot | 79 |
|---|---|
| Maison de vente aux enchères | CHRISTIE'S |
| Date de l'enchère | 10.07.2024 |
Erreur ordre
| Numéro de lot | 79 |
|---|---|
| Maison de vente aux enchères | CHRISTIE'S |
| Date de l'enchère | 10.07.2024 |
Вы уже сделали ставку в € 40 000 и ставка принята аукционным домом. Если вы хотите ее уменьшить, то обратитесь напрямую в администрацию аукционного дома.
Erreur ordre
| Numéro de lot | 79 |
|---|---|
| Maison de vente aux enchères | CHRISTIE'S |
| Date de l'enchère | 10.07.2024 |
Вы не можете делать ставки в данном АД, т.к. были внесены в черный список.
Déposer un ordre
| Numéro de lot | 79 |
|---|---|
| Maison de vente aux enchères | CHRISTIE'S |
| Date de l'enchère | 10.07.2024 |
Autobid est prêt
| Numéro de lot | 79 |
|---|---|
| Maison de vente aux enchères | CHRISTIE'S |
| Date de l'enchère | 10.07.2024 |
